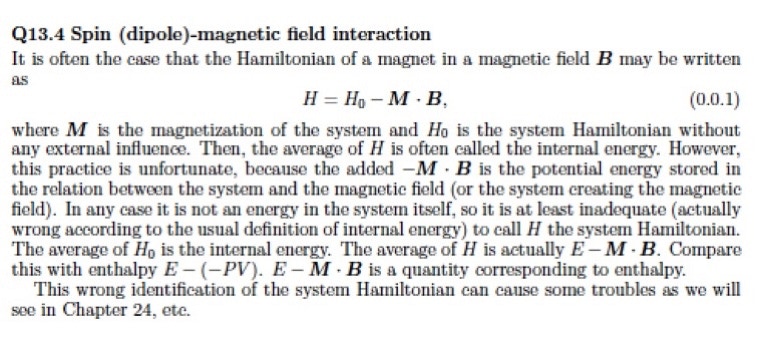
Section 13. Basic Thermodynamics

The domain of thermodynamic coordinates

If we have states that may not be accessed in real physics situations, but if we can be as close as possible to them (i.e., we can be in any neighborhood of these states in the ordinary metric), we must conclude that the domain of the coordinates is (at least on some portion of the boundary) open. 

 Is internal energy continuously differentiable up to the open boundary? Nothing is guaranteed: the limit value and the value at the boundary need not agree (since the boundary is outside the domain). Such a thing could happen at  T = 0; T exactly zero is, strictly speaking, the beyond the reach of thermodynamics, and must be outside its domain as an empirical science.

13.6 A quote from Chapter 14 of C. Bronte, Jane Eyre

``I don't think, sir, you have the right to command me, merely because you are older than I,

or because you have seen more of the world than I have; your claims to superiority depends

on the use you have made of your time and experience.’’

This is a lesson for teachers and for students.

13.6 Prehistory

Let us summarize the time table: